Vibration Isolation and Transmissibility

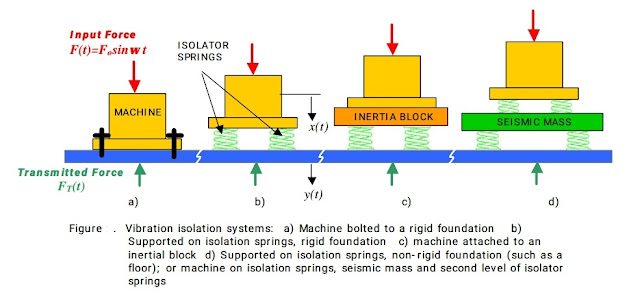
A little consideration will show that when an unbalanced machine is installed on the foundation, it produces vibration in the foundation (supporting structure). These transmitted forces in most instances produce undesirable effects such as noise. In order to prevent these vibrations or to minimise the transmission of force to the foundation, the machines are mounted on springs or dampers or some vibration isolating material, as shown in Fig., The arrangement is assumed to have one degree of freedom, i.e., it can move up and down only.

          An isolation system attempts either to prevent a delicate object from excessive vibration transmitted to it from its supporting structure or to prevent vibratory forces generated by machines from being transmitted to its surroundings. In order to reduce the force transmitted to the support structure, a proper election of the stiffness and damping coefficients must be made.

          It may be noted that when a periodic (i.e simple harmonic) disturbing force F cos ωt is applied to a machine of mass 'm' supported by a spring of stiffness 'S', then the force is transmitted by means of the spring and the damper or dashpot to the fixed support or foundation.

The ratio of the force transmitted (F_T) to the force applied (F) is known as the isolation factor or transmissibility ratio of the spring support.

We have discussed above that the force transmitted to the foundation consists of the following two forces:

1. Spring force or elastic force which is equal to s. x_max and
2. Damping force which is equal to c. ω .x_max.

Since these two forces are perpendicular to one another, as shown in Fig., therefore transmitted,

Transmissibility ratio,

We know that

We have seen previously that the magnification factor,

When the damper is not provided, then c=0, and

From, above, we see that when ω/ω_n>1, ε is negative. This means that there is a phase difference of 180° between the transmitted force and the disturbing force (F cos ωt ). The value of ω/ω_n must be greater than √2 if ε is to be less than 1 and it is the numerical value of , independent of any phase difference between the forces that may exist which is important. It is therefore more convenient to use equation in the following form, i.e., 

Below Fig. shows the graph for different values of damping factor C/Cc to show the variation of transmissibility ratio (ii) against the ratio ω/ω_n.

1. When ω/ω_n= √2 , then all the curves pass through the point ε=1 for all values of damping factor C/Cc .
2. When ω/ω_n < √2  , then ε > 1 for all values of damping factor C/Cc . This means that the force transmitted to the foundation through elastic support is greater than the force applied.
3. When ω/ω_n > √2  , then ε < 1 for all values of damping factor C/Cc . This means that the force transmitted through elastic support is less than the applied force. Thus vibration isolation is possible only in the range of ω/ω_n > √2. 

          We also see from the curves in Fig. that the damping is determined beyond ω/ω_n > √2 and advantageous only in the region . It is thus concluded that for the vibration isolation, dampers need not to be provided but in order to limit resonance amplitude, stops may be provided.    

Vibration Isolation and Transmissibility -- 2 Level model (2 DOF)

          If supporting floor is sufficiently flexible, then two level (or higher) model is more accurate representation. This model also useful for the case where an inertia block (seismic model) is used between the machine and the rigid foundation. 

          If we neglect damping, the motions of the masses, m₁ and m₂ to an input force on the upper mass of  F_EQSinwt  are:

The transmissiblity  (ratio of force transmitted to foundation to the input force), is :

The natural frequencies of the system are the two values of W where the denominator is equal to zero. In the olden days, we would  have factored this equation into the form aw⁴ + bw² + c = 0, then found the two roots using the quadratic equation,

now it is more convenient to put equation 12 into a spread sheet and calculate the transmissibility over a range of frequencies, as in Fig. There are now two natural frequencies, and two frequencies at which the system can resonate. The slope above the second resonance is now -40 db/decade.   

Higher Order Vibration Modes

          In previous topics we assumed a simple translational motion of the machine and its supports in vertical direction. This is some times is called the "bounce" mode. Higher order, rotational modes are also possible, including pitch and roller (Refer Fig.) These modes are not desirable and hopefully are of a lower magnitude than the bounce mode. Proper location of machinery on the isolators can minimise the excitation of these modes. Ideally the machine should be located on its base frame and isolators so that excitation forces act through the centre of gravity of the system, so as not to excite these rotational modes. Large (wide) inertia blocks can also be used to control these modes.